
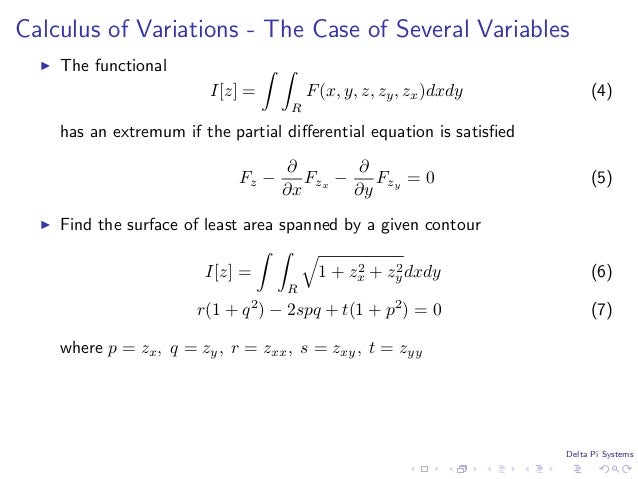
Springer series in computational mathematics, vol 31. Hairer E, Lubich C, Wanner G (2002) Geometric numerical integration, structure-preserving algorithms for ordinary differential equations’. In: International series of numerical mathematics, vol 139. Hager WW (2001) Numerical analysis in optimal control. Int J Control 11:393–407ĭe León M, Martí’n de Diego D, Santamaría-Merino A (2007) Discrete variational integrators and optimal control theory. Found Comput Math 9(2):197–219Ĭadzow JA (1970) Discrete calculus of variations. Numer Math 103:1–10īou-Rabee N, Marsden JE (2009) Hamilton-Pontryagin integrators on Lie groups: introduction and structure-preserving properties. J Geom Mech 5(1):1–38īonnans JF, Laurent-Varin J (2006) Computation of order conditions for symplectic partitioned Runge-Kutta schemes with application to optimal control. J Dyn Control Syst 15(3):307–330īloch AM, Crouch PE, Nordkvist N (2013) Continuous and discrete embedded optimal control problems and their application to the analysis of Clebsch optimal control problems and mechanical systems. Pergamon Press, pp 242–247īloch AM, Hussein I, Leok M, Sanyal AK (2009) Geometric structure-preserving optimal control of a rigid body. This process is experimental and the keywords may be updated as the learning algorithm improves.īock HG, Plitt KJ (1984) A multiple shooting algorithm for direct solution of optimal control problems. These keywords were added by machine and not by the authors. This new geometric understanding of those systems has made possible the construction of suitable numerical techniques for integration.

Recently, in discrete optimal control theory, a great interest has appeared in developing numerical methods to optimally control real mechanical systems, as for instance, autonomous robotic vehicles in natural environments such as robotic arms, spacecrafts, or underwater vehicles.ĭuring the last years, a huge effort has been made for the comprehension of the fundamental geometric structures appearing in dynamical systems, including control systems and optimal control systems. Optimal control theory is a mathematical discipline with innumerable applications in both science and engineering.


 0 kommentar(er)
0 kommentar(er)
